BACK TO ARISTOTLE? 2
B. Lukács
CRIP RMKI, H-1525 Bp. 114. Pf. 49., Budapest, Hungary
lukacs@rmki.kfki.hu
0. FOREWORD TO THE SERIES
Aristotle of Stageira is the Prügelknabe of History of Science since the French Enlightment, c. 1750. As you know, the Prügelknabe is cca. scapegoat; but in a more direct way. Originally the Prügelknabe was a substitute boy beaten if the young son of the Fürst gave a wrong answer to the tutor.
For 250 years Aristotle is beaten instead of (or: together with) the Catholic Church by Historians of Science (h. of sc.). Any ancient Greek scientist other that Aristotle may have told anything: historians of science are still enthusiastic telling that it was originally a congenial idea. Platon may have discussed the Soul which grows wings in ten thousand years and then flees above the sky; h. of sc. tell what a nice allegory. His nephew Speusippus may have written a morally terrible letter to Philip of Macedon; h. of sc. do not discuss this letter telling that it may not be genuine. Speusippus' son, Eurymedon, may have accused Aristotle for asebeia (roughly: a mortal sin against religion), h. of sc. elegantly ignore the event. Only Aristotle has no right to err.
No doubt, this aversion originated from the close ties between Aristotle and the Catholic Church. Since St. Thomas Aquinas, the teachings of the Philosopher were in the fundaments of Church Science. For some centuries almost anything which he had taught was regarded as Truth.
There are old stories, e.g. when a faculty colleague of Albertus Magnus was confronted with a fly. He was told to count the legs. His answer was: if the Philosopher's text were not clear, I should accept that a fly has six legs. (The story is too nice. The Philosopher's texts are not unequivocal about the numbers of legs of the fly. Some years ago my colleague and Aristotle expert, K. Martinás tried with a hypothesis that Aristotle counted the spider's legs and applied the result on the fly by analogy. It is possible: a fly is not only smaller than a spider, but also its legs move much faster. So it is easier to perform the counting on a spider. Remember that the number of human chromosomes was also miscounted up to the 1950's as 24 pairs, which is the correct number for our closest living relatives but not for us.)
St. Thomas Aquinas listed a very few points where he (and so later the Church) did not agree with Aristotle; they were mainly in connection with Man's Immortal Soul. Well, he could not use the gifts of Divine Revelation (albeit Sir Thomas Browne cites an antique author of Jewish religion in Alexandria who claimed Aristotle to have converted). Anyway, Aristotle is an authority in Science for the Church, and continuously ascends in authority. The (posthumous) heresy trial of Wyclif ends in 1415 with the sentence that Wyclif was indeed a heretic; some 260 points of his heresy/errors are condemned and one of them is that he contradicted Aristotle's De lineis insecabilibus. And Pierre Chaunu [1] tells us that Giordano Bruno was burned because he had refused Aristotle's Cosmology. (Indeed, the multiplicity of Suns and Earths is blatantly contrary to Aristotle.)
So, when Enlightened Catholic French freethinkers and anti-Roman Protestants find the Roman Church as common adversary, they attack Aristotle as a symbolic figure. Platon is harmless; he was simply a pagan.
Now, surely our present science is better than the Philosopher's 2350 years ago. Also, the paradigm of the recent physics is different from the Aristotelian one, except in Thermodynamics. (For other sciences, and for social disciplines the differences in paradigms are not so clear.) However, we are just learning that Nature can be described in more than one way. And paradigms cannot be disproven in another paradigm.
Galileo believed he could prove the Copernican system Now we know this would have been an impossible task. In General Relativity you may choose any coordinate system whose origo you consider at rest. In the Ptolemaic ~ Aristotelian description this is the center of Earth, and the (x,y,z) axes are fixed within Earth. With this choice some "inertial forces" appear in the laboratory; the most spectacular continuously changes the plane of the Laplace pendulum ("so proving Earth's rotation", but this is not a proof in the sense of Mathematical Logic). On the other hand, in the Copernican description the center of Sun is the origin. Then the inertial forces in the terrestrial laboratory are much more moderate; however not zero. Two old examples are the tides, well known from the time of Alexander the Great, and the aberration of starlight, observed first by Bradley in XVIIth century.
If you want to use astronomy and physics simultaneously, then you can do it for first approximation more easily in the Copernican paradigm. Without physics if you want to describe the motions of planets, it is simpler in a heliocentric coordinate system, while for the lunar motion a geocentric system is more convenient. But neither of them is "exact, so the true one".
But then, sometimes the Aristotelian paradigm may be useful even in 2007 AD. My colleague, K. Martinás succintly called my attention to this fact some twenty years ago.
Here comes a material from which a Power Point Presentation was extracted and presented at the Hungarian Academy of Sciences on October 15, 2010, at the Simonyi Memorial Conference.
ABOVE PARADIGMS:
THE IDEAS OF SIMONYI, MARTINÁS & LUKÁCS ABOUT THE DYNAMICS OF ARISTOTLE AND NEWTON
B. Lukács
CRIP RMKI H-1525 Bp. 49., Budapest, Hungary
ABSTRACT
Aristotle is the Prügelknabe for many historians of Science in the last quarter millennium, while he was The Philosopher for more than 1600 years. For a physicist this is absurd: while the physics of a scientist in 4th century BC cannot be so good as that of one from 17th AD, Physics is continuous. Based on a chapter of Simonyi’s great book, Martinás suggested me in 1998 a scheme in which a whole continuum of paradigms can be constructed interpolating between the Aristotelian and Newtonian ones. For reasons immaterial here the task was put away for some time but now let us see the first steps.
1. INTRODUCTION
First: do not confuse the persons, because here
Simonyi is not Charles but Károly
Martinás is not Dumitru but Katalin
Lukács is not George but Béla
There are Sciences and Scholarships; scientists and scholars. For the first you may think of, say, Physics, Geology or Entomology; for the second Philosophy or History. The aims and ways of argumentations differ between the two classes, but we often forget this.
Of course, History of Science is a scholarly topic, and indeed often the historian clearly has his preferences, having Heroes vs. Villains. But this is still as interesting as a true novel, if it is well written.
However when History of Science is written by a Scientist, not by a Scholar, then another scientist may learn something about Science; or at least gets ideas to think about.
Simonyi’s book [1] is such a work.
The birth of Newtonian physics is a favourite topic, which, however, is ofgten narrated as a novel or as a political brochure. This is interesting, but a physicist cannot learn anything from it which would be useful in research work. E.g.
According to general opinion (since Enlightment):
Aristotle did not know that bodies continue moving forever without force;
but Galileo & Newton already did know.
and
This is the way how Progress throws ideas to the middenheap of History...
See an example:
2. AN EXAMPLE
Feynman writes somewhere (I read it in Magyar [2] and did not find the English original, so I have to translate back):
"...a múltban arról vitatkoztak, hogy a Föld lehet-e a világ közepe. hogy a Föld mozoghat-e a Nap körül, vagy nyugalomban van. Ennek eredménye borzalmas viszály, sőt háború, és sok bonyodalom."
I.e. approximately:
"...in the past argumentations had happened whether Earth can be in the center of the World, can She move around Sun, or is She at rest. The consequence was horrendous conflict, even war, and many complications."
Of course Feynman does not know either History or Theology; he is a physicist. But maybe he implies Galileo. But the Thirty Year War was not about Galileo's cosmology. Its fundamental cause was the continuous conflict in the German Empire between the leagues of the Catholic and Lutheran Electors, landgraves &c.; the immediate cause was the Bohemian election of Christian of the Palatinate, the Winter King, brother-of-law of James I/VI, King of England/Scotland; and the formally internecine struggle in Papacy when the Spanish cardinals wanted the Pope becoming more involved against the Protestants (really for helping the Spanish & German Hapsburgs) and instead they got only the mock trial of Galileo.
There is a text in Old Testament, Proverbs (of Solomon) 11, 29, whose translations are many, but in KJV: He who troubleth his own house shall inherit the wind…. (In alternate translations: …he sows wind and then reaps tempest…). In our case: if a physicist (or astronomer) initiates ideological argumentations, then he invites professional ideological fighters to decide scientific questions. And then the scientific discussion will be decided unscientifically.
Naturally Simonyi knew this.
3. CONTRARY TO QUASI-HISTORY OF SCIENCE...
Aristotle did know that in vacuo the motion never would stop: Φυσικη ακροασις Book 4, Bk N° 215a14-24 [3]:
"...So that a thing will either be at rest or must be moved ad infinitum, unless something more powerful gets in its way..."
Aristotle did know that he does not yet understand something in the description of ballistics, Μεχανικα 32, Bk N° 858a14-18 [3]:
"...Or is it absurd to discuss such questions, while the principle escapes us?"
Simonyi did know that Aristotle describes the stationary motions in Mechanics, not the transients, [1], p. 61, in my translation:
"...We see that the velocity grows for a while, and then it asymptotically goes to a constant value, v~F/R. This value completely agrees with that given by the Peripatetic dynamics..."
Lukács & Martinás did know that a physicist must be careful to keep the scientific questions strictly scientific, otherwise ideologists transform the physical statements into ideology and then...
Martinás did know that the physical "motion" at Aristotle can be many things in our modern physical terminology, in the majority of cases rather thermodynamic changes, not mechanical motion; and that the physics of Aristotle, with laws of motion of first order, not second, is adequate in Thermodynamics even now. Quoting one of her colloquial private communication: "Aristotle already had one and half of the Three Laws of Thermodynamics"; for more detailed and more professional statements see [4].
Kuhn & Lakatos did know that Truth has strict meaning only within a paradigm. Statements of one paradigm generally seem false viewed from the another one.
Что делать? (Chernyshevskii & Lenin)
4. EXAMPLE: WHAT ORBITS WHAT?
Eudoxus, Aristotle, St. Thomas Aquinas, Tycho Brahe &c: Sun orbits Earth
Arguments e.g.: At Aristotle Earth is composed of heavy matter, therefore were she not around the Center of Gravity, she would tend to reach it. On the other hand Sun is composed from ether or 5th element (quintessence), so Gravity does not affect him. (Περι [BL1]κοσμου, Bk N° 392a5-30 [3]). Concluded anybody from mere astronomical data that Sun occupies the Center and heavy Earth orbits him, he would contradict Physics, especially the facts about Gravity.
Pythagoras, Aristarchus of Samos, Copernicus &c: Earth orbits Sun.
Arguments e.g.: Aristarchus measured Sun's diameter as at least 19 times that of Moon. Then Sun is bigger than Earth. It is absurd to assume that the big orbits the small.
Einstein, 1916: This is simply a matter of choice of coordinates.
Argument: The correct physical description is covariant. Now, covariance means that any coordinate transformation is permitted if the Jacobian does not degenerate. So a geocentric coordinate system is as permitted as a heliocentric one, with an infinite variety of others as well.
No surprise that this last opinion, canonical in General Relativity, also met ideological criticism. See e.g. [5], written in Magyar being originally a dissertation for the degree Doctor of Science (the last below Academician) in 1971:
"Ez az álláspont azonban a feje tetejére állítja a fizika és a geometria valóságos viszonyát."
In my translation:
"This standpoint, however, makes the relation of physics and geometry topsy-turvy."
Two other pearls from the same work, with my translations:
"V. A. Fok ebben az utóbbi kérdésben részben már helyesen bírálja Einstein álláspontját és megvédi vele szemben a gravitációs egyenlet jobb oldalát. Sajnos azonban az egyenlet baloldalának értelmezésében változatlanul megtartja az einsteini álláspontot, ..."
"V. A. Fok's criticism [Russian academician!] in this last question is already partly [!] correct about Einstein's standpoint when defends the right hand side of the gravitational equation against him [against Einstein!]. But unfortunately in the interpretation of the left hand side he [Fok] remains at the Einsteinian standpoint..."
"Íme: a hiposztazált Riemann-tér a maga görbültségével és egyéb meglepő tulajdonságaival, mint a mennyország szálláscsinálója!"
"Look: the hipostasied Riemannian space with its curvature and other amazing properties as the billeting officer of Heaven!"
5. TRUTH AND REALITY; OR: WHAT (THERE) IS?
Obviously the "final" question "what orbits what?" may be final in 2 senses: 1) what is the final answer? (or: have we found it already?); and 2) is there a final answer at all? Obviously the two questions are not equivalent at all: while our answer for the first may not (cannot?) be correct, it is rather difficult to give any serious answer for the second (even if it could be only a simple Yes/No). Since what has been told in the previous few lines blatantly contradicts to "common sense", I should rather explain it.
The "final questions" generally can be formulated in the way: "What is the Truth about...?" In the title of the previous Chapter I wrote "What orbits what?"; but obviously the question is something "What is the Truth about the orbital motions?". Obviously one should define Truth, but this will be put to second place; first, what is the real meaning of "Is"?
One of the most difficult questions of philosophy is the exact meaning of "Is". Maybe the reason is that the meaning is very language-dependent, and the languages were defined ages ago without serious use of Mathematical Logic.
In English everything seems clear; but only because of familiarity. The verb "to be" and its Sg. 3rd Pers. "is" mean several different things. "This spot is green" is not the same construction that "This green spot is on the table", while both statements may be "simple facts". The first is a favourite example of Quine for statements seemingly simple and factual. Indeed, as Quine tells in the Introduction of [6], the situation when we see a green spot and simultaneously we state that "It is a green spot" is rare but it makes the episthemologist happy. I will have a comment in due course even on this; but let us now pass.
"This spot is green" wants to be a statement about the "nature of something", if possible, about the "indelible character". In several cases it is believed "timeless", as "Water boils at 212 F°", expressing chemical (physical?) fact. It stands in Simple Present, but only because it must stand in a Tense. (Of course, even this is not exactly true. The boiling point depends on the atmospheric pressure.) In this sense "This spot is green" may mean "This spot is green by its indelible nature"; but it may also mean "This spot is green now, but will be blue tomorrow". Not quite the same. On the contrary, "This green spot is on the table" does not tell anything about the indelible nature of the spot. The spot is now on the table; it could be anywhere else.
Now about language-dependence, which is important, because "Final Truths" should not be language-dependent. However making the statements in Magyar:
"This spot is green" = "E (This) folt (spot) zöld (green)"; while
"This green spot is on the table" = "E (This) zöld (green) folt (spot) az (the) asztalon (table-on) van (is)"
In the first statement about the nature of the spot there is no verb at all. The sentence "E folt van zöld" is completely impossible in Magyar; the sentence "E folt van" states the existence (more or less timeless), while "E folt az asztalon van" states the location of the existing spot, either timeless or temporary. Going further in languages, in Japanese there are two (or more) verbs with parts of the meaning of "is": arimasu/imasu is "to be somewhere" while "desu" is "to be something". In Turkish, very far from English but not exactly so far from Magyar "the 'is' of nature" is not even a verb but a suffix:
"Türküm" = "Türk-üm" = (I) am Turkish"
and this suffix can be used also to state locality only with slight differences of emphasis. "Iron is heavy" is "Demir (Iron) ağırdır" (heavy-is)" and "He is Turkish" may be "Türkdür". However -dir/-dır/-dür/-dur is not the "verb of existence". It is a suffix, very near to the personal suffix of verbs in 3rd Sg. But note that "Ahmed is at home" is "Ahmet evde (home-at); "Ahmet evdedir" is rather "Ahmed must be at home". And -dir can supersuffix verbs in 1st or 2nd persons: "Görmüşsünüzdür" is cca. "I expect you have seen", where "Görmüş" is cca. the "narrative past" of "to see", "sünüz" is the Pl. 2nd suffix, and "-dür" here means "I expext/it is expected/it is sure". (For more see e.g. [7].)
I think these examples illustrate that "It is" is not quite a scientific term. Now, "is" may be substituted with "exists" in some case (even if Quine preferred “is” [8]), and we can tell that "The green spot exists in the table"; and then the existence is nicely distinguished from the other "is" in "This spot is green", but still we do not know if the green spot "exists" on the table always, or just now. Well, one could tell in the second case that "the green spot is existing on the table", but it is generally not done.
At this point the obvious solution would seem to be: "use symbols of Mathematical Logic instead of everyday language". Indeed, I suggest this, but for that Mathematical Logic should take seriously. Before this see the postponed question: "Is there a final answer at all?" What would confirm the Final Answer? If nothing, then why to speak about it?
This problem is very, very philosophical. However a famous psychologist, Julian Jaynes, gave a strange answer to the question "Why we look for such answers?" While I am not arguing for his answer, it is worthwhile to cite it [9].
The scheme told in the book is provocative, and very briefly it tells that the human mind even in historical times worked differently from the present mode. The two hemispheres are quite autonomous even now, and Jaynes believed to see signals that the autonomy was much more complete in Neolithic and Bronze Age. Then the left hemisphere got ideas from the right one only in rare occasions of "crises", i.e. in situations when "direct", "step-by-step" thinking reached its boundaries. Then the right hemisphere took over and gave a command. Now this command of course may or may not have been useful, but it was no worse than having no idea at all.
Of course the respective humans did not know that they had hemispheres: the left brain was "they", and the right one was "a god". Intuition was believed divine. Jaynes illustrated his scheme with lots of citations from the Iliad, where practically nobody himself decides or invents anything; in all new situations commands come from divine persons, e.g. Odysseus generally gets them from Athena. Now, in the Odyssey, somewhat later according to most authorities, Odysseus sometimes got ideas from himself but the other guys do not. In later Greek thinkers, ideas generally come from themselves, although Socrates had his daimon; and divine intuition goes to oracles. As Jaynes writes [9]: "...In the second millennium B.C., we stopped hearing the voices of gods. In the first millennium B.C., those of us who still heard the voices, our oracles and prophets, they too died away. In the first millennium A.D., it is their sayings and hearings preserved in sacred texts trough which we obeyed our lost divinities. And in the second millennium A.D., these writings lose their authority. The Scientific Revolution turns us away from the older sayings to discover the lost authorization in Nature. ... Science then ... is not unlike ... the same nostalgia for the Final Answer, the One Truth, the Single Cause. ... And this essay is no exception."
Now, of course, this interpretation is vanitatum vanitas. OK, maybe everything is vanitatum vanitas, maybe Life has No Meaning, and we might peacefully contemplate. But maybe not. Good Science is useful, as a great number of examples show it. Let me cite Jaynes once more: We, we fragile human species at the end of the second millennium A.D., we must become our own authorization."
Do we know, how? But let us deliberately forget about Final Truth, and let us try to make Useful Physics. Physics can be formulated in many paradigms. And what is the relation amongst them?
6. THE TRUTH ACCORDING TO QUINE
In the previous Chapter I cited some ideas of Quine. This Chapter is centered on him. He worked on Mathematical Logic, and note that Mathematical Logic is the direct descendant of Peripatetic Logic, founded by Aristotle himself.
Of course, the beginnings of Mathematical Logic are older than Aristotle. The first philosopher we know about, who tried to formulate rules of statements not absolutely rubbish for us is Socrates. His teaching was communicated by Plato's Academy, where Aristotle was a young teacher. But afterwards the Aristotelian/Peripatetic science evolved divergently from Platonism.
And the Peripatetic tradition is continuous. Plato's Academy was closed in 529 AD by Justinian, and "Platonism" was revived from old books in Rinascimento, but in Late Antiquity the Peripatetic School had both Pagan and Christian philosophers, and in Middle Ages both Christian and Muslim followers (both Sunnies and Shiites) [10]. Abelard and Petrus Hispanus were as true followers of the Peripatetic founders as were Avicenna or al-Suhrawardi. And this useful science continuously evolved into Scholastics, and then into our Mathematical Logic. And this was possible because the scientists were free to choose between paradigms, and then chose the most useful. (Abelard was not castrated by Church for using Peripatetic paradigm; the act was performed for the money of a canon whose niece Abelard had seduced. True, he later married Heloise, but did not make the marriage public, so the canon felt to be in shame.)
Now let us follow Quine [6], [8], [11]. We have experience about the external world. Because Peripatetics are not visionaries, these experiences come from observations/perceptions. Our mind gets external data; however it uses hypotheses in evaluating them. The scheme is illustrated by Fig. 1.
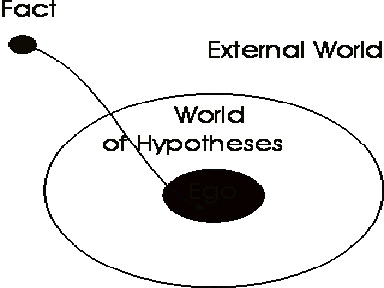
Fig. 1: Quine's scheme of observing World. The "facts" are observed through the filter of our hypotheses, so no "direct fact" is known (or, at the best, a very few).
As an example, take the situation when the observer observes a green spot of light. Then he may say: "I see a green spot", and such a clear situation is rare but makes the episthemologist happy [6]. (My comment expanding beyond Quine will come.) But: may he conclude that the surface whence the light was reflected is green?
He may; but Quine calls attention to the hypotheses made here. E.g. the rules of reflection from a surface are hypotheses. Also: you may assume that the wavelengths change during propagation: then the surface is maybe blue, but when the light reaches me it is already green. And so on.
I add that such rigorous discussion may seem hypercritical, but people of General Relativity (as e.g. I) do know situations similar to the seemingly absurd one just mentioned. E.g. there is a general "reddening" of lights coming from extragalactic distances. The increase of wavelengths seems cca. 10 % on 1 billion lightyears; but the interpretation is manifold.
The oldest idea from the 1920's was Doppler shift because of velocities away from us ("the recession of galaxies", the term is used even now). Of course, an explanation would be needed, why every galaxy is receding, the farther ones more rapidly.
Then came a General Relativistic explanation. The galaxies do not move too much, but the metric of the space-time is changing via the Einstein Equation, and one of the two simplest/most symmetric solution is "expanding" (distances are growing). Hence comes an "expansion" of waveforms, so reddening.
However either for defence of "common sense" or for other problems alternate hypotheses are still alive. One is an interaction with Something while propagating: one would expect rather energy loss in an interaction, so reddening of the photon, as we indeed "see". The explanation is complicated because the optical images of far sources are not diffuse enough for simple scattering; but not impossible. The other idea comes from Hoyle. Assume that Physics if time-dependent, e.g. in oscillating form, and Something governing atomic energies (say, the “constant” e) was 0 at 15 billion years ago. Then old photons were produced with small energies, so they are red.
And so on...
As for the seeming greenness of a spot, which so far seemed to be a fact (the greenness of the spot does depend on hypotheses about light propagation, but the detected greenness does not) let me list 3 notes very briefly:
1) The colour is a product of our brain and the brain tries to determine something belonging to the reflecting surface, not to the incoming light [12]. This causes the colour constancy under wide variances of illumination.
2) Colour is determined in the brain from signals starting in the retina; in a very limited number of different kinds of sensitive cones & rods. Physically there is no such thing as colour.
3) While colour vision is fairly uniform in human population, it is not absolutely such. It seems that roughly 10 % of men and 1 % of women "see the colours otherwise". The most frequent difference is an abnormal opsin, causing slightly different impressions with a lower colour constancy, but a minority of the minority is Daltonian, with the lack of one kind of cones. Now if he lacks the green one, he "cannot see" green at all, even he believes he sees it, as it happened (vice versa) to Dalton. His Daltonism was discovered when he entered his first fox-hunt in a green tuxedo, seeing it red.
The tetrachromats are even more interesting for the present topics. They exist, although not among men, and they are rarely observed (even by themselves). They are relegated to an Appendix; but note that a green tetrachromat sees two qualitatively different colours in the range where we see one. Then what does her statement means "I see a green light"?
In Japanese our range of blue+green is covered also by two terms: aoi+midori. However, midori is only our yellowish green, or warm green, roughly "golden green". Explicit green, blue-green and blue are aoi, and traffic lights are generally cherry red, yellow & blue-green, giving better discrimination for Daltonians. Now, an elementary ontologic fact should survive translation.
Dogs have no green cones (nor reds); they have shortwave blues vs. longwave yellows. They see the grass yellow. So much about elementary facts.
Now back to Quine. One scientist, A, elaborates a group of coherent hypotheses, called a paradigm. A is then interpreting the further observations using these hypotheses, so in this paradigm. Now a competitor, B, may elaborate another bunch of hypotheses, and may use a concurrent paradigm.
Scientist A is famous enough to propagate Paradigm A. It comes to general use, but some strange results are obtained when evaluating a few observations. To "explain" them too some extra/new/modified hypotheses are needed: they are elaborated, but the whole coherent paradigm is still valid. [An example: in the Aristotelian paradigm celestial bodies move on circles, and the most natural point for the centers of these circles was the center of the Universe, coinciding with the center of Earth. So Aristotle assumed this; but it was not necessary. So when Ptolemy observed changing apparent sizes/angular velocities, he removed the centers of orbits from the center of Earth ("excentricity"), and still he was in the Aristotelian paradigm.
This continuous improvement goes until it is economic. When the necessary effort of rearranging the hypotheses within the original paradigm is already too great, then the paradigm becomes abandoned. Scientists take other paradigms, one is the simplest to reconcile with the known observation, so that paradigm takes over after some argumentation.
Quine suggests something such as the normal way of scientific progress, and this does not contradict anything be seem to know. (Note the appearance of the idea in the 40’s in a Hungarian dystopia [13]; see a very short excerpt in App. A.) Of course, if forces outside of Science act, the normal evolution may be disturbed; but according to our knowledge about History of Science such influences are temporary.
And then Jaynes might be satisfied too. We can speak about rational scientific evolution even without referring Final/Absolute Truth, which we would not recognise even if a Divinity showed us. And no authorization is needed. Only "true" is not a good term; instead "truer" might be used meaning "giving a possibility for simpler description".
7. CONTINUA OF PARADIGMS
Here I refer a project 11 years old and not yet ready. The project was proposed in an email of Martinás to Lukács, 1999 [14], very, very briefly summarized here as:
A continuum of paradigms so that one end is Newtonian physics, the other is the Aristotelian one is possible. We need only one new term. Let us create this continuum!"
I sent several answers about the cooperation for years; all were ignored. So now I do something alone as "a one-handed giant" (cited from L. Kossuth, politician in the middle of 19th century). But, being alone, I make here only the first step. For a continuum of paradigms in another case see [15].
8. VARIATIONAL PRINCIPLE
In mechanics, the (Newtonian) equations of motion are of second order, and they can be got from a Lagrangian:
L = L(x, ∂x/∂t, t)
Hence the equations of motion:
d/dt{∂L/∂(∂x/∂t)} - ∂L/∂x = 0
For simplicity's sake consider a linear harmonic oscillator:
md2x/dt2 + Dx = 0
Its Lagrangian is:
LN = (m/2)(dx/dt)2 - (D/2)x2
Now take the continuous series of Lagrangians
L(K) = LN*eKt
Then the equation of motion is
md2x/dt2 + Kdx/dt + Dx = 0
As for K, it has a form of friction. Maybe "it is real". Maybe not, "we only introduced it". Maybe a part of it "is real", but "we only introduced" the other part.
In the simplest way of Mechanics, i.e. when the whole Equation of Motion is got from the Lagrangian, K=0 is Newtonian physics, and the limit K→∞ is the Aristotelian one. But one may create a Physics where Mechanics has an Universal Anti-Friction (an analogy is Krauss' 3rd Force [16] interpreting Galileo's gravity experiments benevolently), but in Physics K≠0. This is the Aristotelian paradigm where the Universe has a Prime Mover at the very periphery.
This concept is most explicit in Περι [BL2]κοσμου, Bk N° 397b10 - 401b29, [3] but there the language is pseudo-theological, towards which modern physicists have scarce empathy. However note that such a Force can counteract Heat Death [4], and Heat Death in an infinitely old Universe was an unsolved problem for Newtonian physics.
9. ON THERMODYNAMICS AND ECONOMICS
Thermodynamics is based on first order (conduction) equations even now, so it stands apart in Modern (on Newtonian) Physics. Simonyi tells the story from misty pre-Thermodynamics through Scottish engineers to Count Rumford, Sadi Carnot and entropy [1]. And Martinás traces back Thermodynamics to Aristotle [4]; and Aristotelian physical formalisms are of course of first order, as noted by Simonyi [1].
Scientists try to make Thermodynamics second order; and also try to get its fundamental equations from a variation principle. Neither effort is quite successful and the two problems go back almost a common cause: equations obtained from a Lagrangian tend to be of second order as seen in Chap. 8.
Now, the search for the thermodynamic variation method is a duty belonging to Dr. Martinás, so I rather show an analogy, quite actual at the tail of the Big Credit Crisis, namely Economics. Since an interesting result of J. Kovács & Ildikó Virág in 1981 (in Közg. Szemle, Vol. 28, p. 675) we know that a one-sector Harrod-Domar model economy with constant efficiency of capital yields maximal consumption (so e.g. well-being) on definitely non-uniform investment paths. Indeed, that oversimplified model yields it on investments of step-function shape. These mathematical facts may be behind economical cycles, overconsumptions and overinvestments. The society of course wants to maximize well-being.
Now, let us keep all the oversimlifications except that the efficiency of capital now depends on the investment rate s:
Y(t) = g(s(t))K(t)
C(t) = (1-s(t))Y(t)
dK/dt = s(t)Y(t) - λK(t)
(λ being the amortization) and then the maximum of the well-being is the maximum of the integral of consumption C(t) for a fixed interval T.
In the 80's & 90's Dr. Banai and myself published a lot of papers (with a small enough impact) about this problem, see e.g. [17]-[22]. Do not be afraid: I will not go into the details. I only state that the integrated consumption will be the Lagrangian (since we look for its maximum), and by introducing the variable y(t) via the definition
dy/dt ≡ s(t)g(s(t)) - λ
the Lagrangian gets the form
L = F(dy/dt)ey
where, I think, you are not interested in the exact form of F. And then the variational principle gives the equation of motion
{F''d2y/dt2 + F'dy/dt - F}ey = 0
So y cancels, there remain only dy/dt and d2y/dt2, and the final equation is of first order in s(t).
So something is Aristotelian if you like so and still Newtonian/Lagrangian if we want and work for it.
10. CONCLUSION
1) (Hungarian background is needed here to understand.) Famous humorist Hofi telling repeatedly "Egyedül nem megy!", cca. "It does not go alone!" is not true. It goes; only more slowly.
2) I think Quine's ideal can be approached (even if in [8] he seems somewhat dogmatic about existence/non-existence of not quite material individual; Aristotle was more elegant in Bk N° 89b31-35 [2] about gods & centaurs), and Jaynes' ideal is viable too (independently of the historical role of the right hemisphere). Science can be done even without explicitly referring to Final Truth & such practically inoperative entities. A better theory explains easier the important observations, therefore will give more good predictions per manyear than the others for a while, resulting in new inventions, more PhD degrees and higher standard of life. When it is no more true, free researchers in free Science choose a better-working paradigm without heaping indignities on the builder of the previous one. Of course this is possible only if the new paradigm is not denounced being contrary to Bible as in the case of Galileo, or being billeting officer of Heaven as the Riemannian geometry in [5].
3) And see [23] where the Quine construction of Fig. 1 was used in the prediction of hadronic yields from QG plasma.
Science is science so should be made and regarded so.
APPENDIX A: ON TETRACHROMATS
From times immemorial rumours were heard about "richer colour perception" of exceptional people. From c. 1990 human tetrachromacy is well established even if the details are not yet clear. You can find the 1999 state of art in [24]; that will be enough even if the last decade brought new knowledge too. If you does not believe something, check in [24] and the citations therein; let me continue.
Tetrachromacy may happen, if 1) rods' signals enter the cerebral colour determination (a fourth signal) or if 2) there are 4 cone opsins instead of 3. This is quite possible because the red and green human opsins do have frequent variants; but, they being on Chromosome X, the effect is rather expected in women. Indeed, so far only female tetrachromats have been reported.
However, feminine tradition about colour naming, colour handling, social role of colours &c. is rather intuitive, and my experience is that for me it is very difficult to discuss the details of colours with women; sometimes they seem definitel embarrassed. However woman physicists are sometimes cooperative.
One of my colleagues is a green tetrachromat, distinguishing the two colours in my green range when speaking with me; as far as I know she does not use the terms too much when speaking with others (embarrassment?). One is leaf-green (or grass-green; I would call it "grassy"), so cca. the colour of chlorophylle. The other is "fence-green" or "fency", because a paint of this colour is often applied on garden-fences. Her extra cones have the maximal sensitivity at 513 mμ (measured). This is bluish green for me and indeed I see the fences bluish green; but she is unable to mix fency from grassy and blue. The only plant she reported as fency was a stagnant pond with algae; they must have been bluegreen algae, Cyanophyta. And she told me more than once that "colour TV is boring, not having ever fency". Indeed, colour TV uses my green.
Now, what does it mean in Mathematical Logic if she states "I see a green spot"?
APPENDIX B: EXCRRPT FROM KŐ KÖVÖN...
This is [13]; the title means cca. „Razed to the Ground”. An extreme terrorist starts a one-man Crusade against Technical Civilisation with his invention, a liquid evaporating the metals. Of course the novel ends with the tribulations of the last humans, but this is pointless for us. At the beginning the complete overnight disappearance of a bridge is a mystery and journalists interview the President of the Royal Academy because „Publicity needs Truth”. The scientist becomes very angry:
„Long since heard I such a nonsense! What bosh is your Truth!? How you dare to use words about whose meaning you have not the least idea!? Do you think that Science works with Truths? Behold; It does not! There is even no need for Truths! ... Go to Courts for Truths! ... Science walks on hypotheses as crutches ... applicable to lots of situations conveniently and easily. But if we meet inexplicable situations, then we must look for new hypotheses as I must buy new breeches if the old wear out!”
Of course such a consequent and clear formulation appears rarely.
IRODALOM
[1] Simonyi K.: A fizika kultúrtörténete, Gondolat, Budapest, 1981
[2] R. P. Feynman: A tudomány és a vallás viszonya. Term. Vil. 124, 175 (1993)
[3] Aristotle: The Complete Works of Aristotle, ed. by J. Barnes, Princeton University Press, Princeton, 1995
[4] K. Martinás: Aristotelian Thermodynamics. In: K. Martinás, L. Ropolyi & P. Szegedi (eds.): Thermodynamics: History and Philosophy. World Scientific, Singapore, 1991
[5] Elek T.: Marxizmus és relativitáselmélet. Akadémiai Kiadó, Budapest, 1973
[6] W. V. O. Quine: Methods of Logic. Holt, Rinehart and Winston, New York, 1963
[7] G. L. Lewis: Teach Yourself Books. Turkish. English Universities Press, London, 1953
[8] J. Jaynes: The Origin of Consciousness in the Breakdown of the Bicameral Mind. Houghton Mifflin, Boston, 1976
[9] M. Maróth: Aristoteléstől Avicennáig. Akadémiai Kiadó, Budapest, 1983
[10] W. V. O. Quine: On What There Is. Review of Metaphysics, 2, 21 (1948)
[11] W. V. O. Quine: Two Dogmas of Empiricism. The Philosophical Review 60, 20 (1951)
[12] J. Weinberg: The Geometry of Colors. Gen. Rel. Grav. 7, 135 (1976)
[13] Feleki L.: Kő kövön... Magyar Téka, Budapest, s.d.
[14] K. Martinás: Private communication, e-mail to B. Lukács, on May 8, 1998, 14h 33m 20s, subject: thermo
[15] B. Lukács & K. Martinás: Callen’s Postulates and the Riemannian Space of Thermodynamic States, Phys. Lett. 114A, 306 (1986)
[16] L. M. Krauss: The Fifth Force Farce. Physics Today 61, 53 (2008)
[17] Banai M. & Lukács B.: Variációs elvek és mozgásegyenletek. Fiz. Szemle XXXVII, 337 (1987)
[18] Banai M. & Lukács B.: Közgazdasági példák egzaktul megoldható variációs problémákra. Mat. Lapok 34, 307 (1991)
[19] Banai M. & Lukács B.: Beruházási pálya és variációs módszerek. Közg. Szemle XXXIV, 432 (1987)
[20] Banai M. & Lukács B.: Fogyasztásnövekedés, gesztációs és szabályozási késés. Közg. Szemle XXXV, 1307 (1988)
[21] M. Banai & B. Lukács: Optimal Investment Strategy by Variational Principles. KFKI-1989-64
[22] M. Banai & B. Lukács: Attempts at Closing Up by Long Rasnge Regulators. In J. Kovács (ed.): Technological Lag and Intellectual Background: Problems of Transition in East Central Europe. Darthmouth, Aldershot, 1995, p. 311
[23] B. Lukács: Hadron Yields, Physical Reality and the Objective External World. In Budapest 2002 Workshop on Quark and Hadron Dynamics, eds. Judit Németh, I. Lovas & J. Zimányi, EP Systema, Debrecen, 2002, p. 309
[24] K. R. Gegenfurtner & L. T. Sharpe (eds.): Color Vision. Cambridge University Press, Cambridge, 1999